In this presentation, we will take a look at present value formulas related to bonds. One of the reasons bonds is so important to accounting and finance is because they’re a good example of the term of present value of money. We’re trying to look for an equal measure of money, when we think of bonds and bonds is going to have this relationship between market rates and the stated rate, which helps us to kind of look through and figure out these types of concepts. So even if we don’t work with bonds, in other words, if we’re not planning on issuing bonds, or buying bonds, or knowing anything about bonds not being important to us, the time value of money is a very important concept and bonds is going to be a major tool to help us with that. Why is bonds so useful for learning time value of money, because there’s two types of cash flows with bonds meaning at the end of the time period, we typically are going to get the face amount of the bond that 100,000 similar to a note and then we’ve got the interest payments that are going to happen on a periodic To basis, and therefore we have these two different types of cash flows, that we can use two different formulas for, to think about how to equalize.
01:09
So note, when we think about time value of money, what we’re doing is we’re just basically saying that we want a unit of measure, that’s what money is a unit of measure, we’re trying to measure value. But unlike any other unit of measure, most others, like a ruler, the time value of money, or the dollar, the value of the dollar changes, so our ruler kind of changes in size as we’re trying to measure value with it. And that would be similar to our ruler, changing in size when we’re trying to measure how big a table is. So it changes with time. So therefore, if for us to really measure something, we have to know what time period we’re in what time frame are we in? So when we consider something we have to say, Well, here’s the value of money at this certain time period. So in terms of a bond thing, we have two types of cash flows. One, we have the 100,000 and we’re going to say that it’s going to be doing this case at the end of For time periods why, because it’s a it’s a two year bond. And we’re going to say it’s semiannual payments. So that means it’s going to be four periods out, not years, but four periods. And then we’re going to have the amount of interest that we’re going to pay on a semi annual basis. And that’ll happen periodically. So we’ve got the 4000 interest happening each of those four time periods. So we have two types of Cash Flows happen in here, we’ve got we’re going to pay out the 100,000 at the end, and we’re going to pay out 4000. each pay period that 4000 being calculated as the 100,000 times the stated rate, the amount on the bond point oh eight, that would be for a year divided by two because it’s semi annual 4000 each each period or each six months.
02:53
Those are going to be our two flows that we have. Now our question, here’s what really is the value of the bond and we typically Want to know the value of the bond now, today, not at the end of the bond. So we don’t want to know what the cash flow is, that would be easy for us to calculate, it’s going to be the 4000 plus the 4000, plus the 4000, close to 4000, plus the 100,000. That’s how much cash is going to go out. But the units of cash differ, the value of cash differs depending on what time frame we are in. In other words, this 4000 a year from now is worth less than 4000. Today, this 4000 this would be six months from now 4000 a year from now would be worth less than 4000. Today, 4000. A year and a half would be worth less and this two years out that we’re going to get the hundred thousand and the 4000 is going to be worth less than 104,000. In other words, we would rather have 104 today, why? There’s two there’s two factors. One is that we know that there’s interest so that the value of purchasing power will actually go down.
04:00
And to there’s opportunity cost, we could have put, if we had that money now we could put it somewhere else. And therefore, the fact that we could put money to work means that it’s worth more today than in the future. So for those reasons, we got it, we got to present value this information, we have to take these cash flows in somehow put it into the present value. Now, there’s two types of ways we can do that one, we could try to take each number and bring it back to the present. And we will do that with this 100,000 because there’s only one payment, which is two years out or four payments out. So we’re just going to take that hundred thousand in present value it but here we’d have to take the 4000 which is one period out or six months in present value it and then present value this 4000 which would be a little bit different. This is going to be worth a little bit less in terms of present value dollars, because it’s a year out or two time periods. And then this is a year and a half out it’s going to be worth a little bit less than this 4000 so because they’re all the same We call that an annuity, the payments going to be the same, we can simplify the formula and use an annuity type of formula to do that. There’s a couple ways we can do this in practice. So we can do this with a formula.
05:12
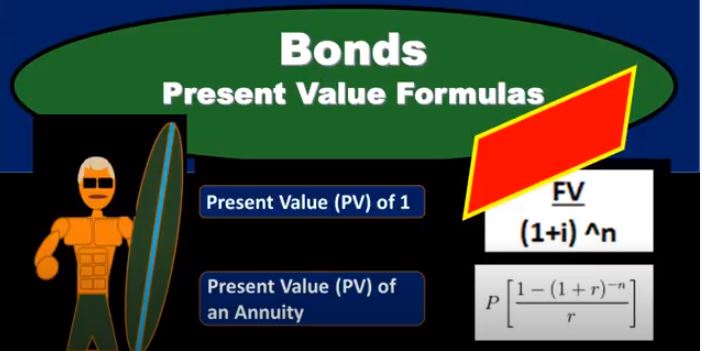
We can do this with Excel, we can do this with a financial calculator, or we can do it with tables, just depends on the course on how you, you know how they display that and what tools you have to work with it. Clearly, to me, Excel is probably the easiest thing to use or financial calculator, the formulas, what we want to start with, however, in order to see how these are kind of derived, what’s the work doing, so we won’t try to derive the formula we’ll just show you here’s the formula that we will be working with. So you could if you’re in a situation where you have to just work with a formula, you can memorize the formula. If you’re obviously working in practice, then you’re going to be using most likely Excel or a calculator to do this. We’ll look at it with a formula first and then look at the other tools. Note of course what we’re doing. What you want to know is conceptually what is happening. Why are we doing this? What does it mean? What can it tell you. So here’s going to be our present value formula, we’re going to take the future value divided by one plus the rate or interest to an, which is going to be the number of time and then the present value for an annuity, it’s going to be a bit more complicated. We’re going to take p or the value of the bonds, the payments, and then we’re going to take one minus one plus r to the negative n over the rate. So this would be the math way to do it. Again, we could kind of shortcut this with a calculator or Excel, but let’s look or tables. So let’s look at the concepts of this. So we’re going to take this formula, we’re going to plug our information into this formula, present value, often recorded as PV of one is kind of a formula that we are doing here.
06:52
We’re going to apply this more simplified formula just to the face amount that we’re going to pay out if we’re issuing the bond and we’re going to pay At the end, we’re going to pay it out at the end of the time period, the 100,000, in this case being four time periods, because it’s two years that we’re going to pay this out two years in the future. And it’s paid semi annually. So if we plug that into our formula, then we’re just going to say that the future value is 100,000. What does that mean future value, it means that this 100,000 is how much we’re going to pay out after four time periods at the end of two years. But that’s future value. It’s not It’s not what it’s worth right now. That’s how much we’re actually going to pay in dollars, but it’s not the value now today. So that’s the future value. If we divide that by we’re going to plug the rest in one plus point oh five. Where does that come from? Well, we have a market rate, which we’re going to use for this calculation, not the stated rate. We’re gonna use the market rate because that’s the that’s the market rate. And we’re going to divide it by two because we have the the rate is per year. We want to make it per six months. So we’re going to take that market rate if we move the decimal over 10% divided by 2.05. And then we’re going to take that out to the number of periods for two years, there’s two payments per year. So this is our our math that we’ll have to work out. Now, I recommend not doing this in Excel if you’re going to do it like a formula here, because it’s easier to write it in paper. Even if you have Excel, it’s often easier to write something like this in paper and just do the algebra meaning do one step at a time, we’re going to add the you know, the one plus point oh five is 100,000 divided by 1.05 to the fourth, and then we’re going to take the 100,000. We if we do the math here, we got 1.216 rounded, and that’ll finally get to the 82,002 70. That means that this 100,000 that we’re going to pay out two years from now or four time periods is worth present value in today’s dollars, only 82,230 70.
09:00
So if this was the only thing that was happening, meaning we’re going to pay out 100,000 in the future and there was no interest involved, then we would expect 82,272 day in order for that to be a fair transaction. But that’s not the only thing that’s happening. We’re also paying out interest. So let’s do the same thing for the interest calculation. Now, we could do it one by one, we could take the interest for one six month period, the second six month period, but it’s easier to use an annuity, maybe not with this formula. But if we have a Excel or a calculator or tables, the annuity table will be faster than calculating each payment, especially if it was a long annuity. So this is going to because although they’re all the same, we can use this formula to calculate the annuity. So we’re going to take the 4000 then we’re just going to plug this information into our our formula times. That’s P times one minus one plus point O five Same point oh five market rate divided by two to the negative four y negative, we’re just going to flip the sign, basically, we put in a negative to flip the sign of this final result. And then we’re going to divide it by point oh five, the rate once again, 10% divided by two. That’s our formula. If we just do the math, algebraically, write it down to do the math, I wouldn’t recommend doing it in Excel, you want to write it down to one step at a time. So we’re going to add up these inner columns, one minus point five to the n to the negative four. And then we’ll do the math here and get the point one eight, rounded, divided by point 05, times the 4000, of course in both of these, and then if we finish up the math, we’re going to say 4000 times 3.55, dividing this out, and that of course, will be the 14 184. So note what this means that we had four payments of 4000, meaning we’re going to pay 100 hundred thousand times the point oh eight, that’s somewhat divided by two.
11:10
That’s how much we’re actually going to pay four times times four. So we’re actually going to pay 16,000. At the end of this time period, we’re going to pay 4000 every six months, four times. But it’s not really worth 16,000 it’s worth 14,184. So if the only thing we were doing here, if we’re weren’t paying 100,000, and we were just gonna pay back 4000 each year, each six months for for two years or four time periods, we would expect 14,001 64 in today, in order to make those payments in order to have a fair transaction and negotiable, marketable transaction. Note when we calculated the interest of 4000, we use what we’re actually You’re going to pay, when we use this calculation, we’re going to use the market rate. Because that’s what the market is doing at this point in time. So if we if we add those up, then the, we’re going to pay back 100,000 at the end of the time period, that’s worth 82 to 70 today, and then we’re going to pay 4004 times every six months for two years for two years, that has a current present value of 14,001 84 for a total of 96 for 54. Therefore, if we were to issue this, this bond for 100,000, we’re going to say we want money today, we’re going to give you 100,000 at the end of two years, and we’re going to give you 4000 every six months for that two year time period. How much would we want for it today? We want 96,454 according to the market rate of 10% at this time, therefore the journal entry is would just be that cash is going to be that 96 For 54 that we just calculated, the bond is going on the books for 100,000. And then we’d have the discount of 3005 46. So cash would be going up, that’s why we issued the bond, the bonds going on the books with 100,000 that we owe, and then we discounted it by the 3005 46 in order to equate it to the market value. So this credit what we owe minus the discount is the carrying value of the book value of the bond